Medical Test Result

He was in shock.

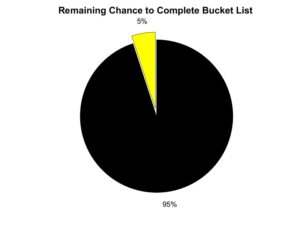
The doctor told him of his positive screening result. “This test detects 95% of all prostate cancers.”

He muttered. “I’ve got cancer.”

“Maybe. Maybe not. I need further information to make that diagnosis.”

“You need more than 95%!” He shook his head in wonder.

The doctor sat and explained further. “No. If you have prostate cancer, the test will detect it and give a positive result 95% of the time. That’s the sensitivity of the screening test. There are 5% who have it but were not detected by the test. We called that the False Negative Rate.

“If you don’t have it, the test will be negative 94% of the time. That’s called the test specificity. You could be among the 6% people without prostate cancer who get a False Positive.”

“That doesn’t make me happier. I only have a 6% chance of not having this cancer.”

The doctor listened calmly, then told him. “There are more factors at work. Only 12% of men develop prostate cancer in their lives. You enjoy mathematics, don’t you? Well, Bayes Theorem comes into play. It adjusts the probability that you have the cancer given that you tested positive by considering the sensitivity and specificity of the test.

“The probability you have prostate cancer is the probability of your positive screening given you have the cancer divided by the probability of all the ways you can get a positive screening result.”

He tapped on his medical tablet, then turned the screen toward his patient.

Facts:

(1) $\begin{align*} P(C) &= 0.12 \text { Probability that a man has prostate cancer.} \\ P(+|C) &= 0.95 \text { Probability of a positive screening test when man has the cancer.} \\ P(+|~C) &= 0.06 \text { Probability of a positive screening test when the man does not have cancer.} \end{align*}$

Determine Likelihood of Prostate Cancer: P(C|+). The probability the patient has cancer given the patient tested positive.

(2) $\begin{align*} P(C|+) &= \frac{P(+|C)*P(C)}{P(+|C)*P(C) + P(+|~C)*P(~C)} \\ P(C|+) &= \frac{.95*.12}{.95*.12 + .06*.88} \\ P(C|+) &= 0.68 \end{align*}$

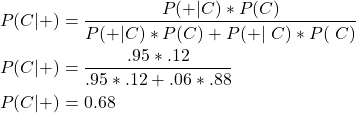
The patient with a positive prostate screening and no other symptoms has a probability of 68% of having the cancer.

Recommendation: Order a PSA blood workup. Check for family history of prostate cancer.

Reasoning: Among 100 men, 88 men who won’t develop prostate cancer will contribute 5 false positive results (88*.06). The 12 men who do develop prostate cancer will contribute 11 true positive results (12*.95). Of 16 total positive results, only 11 are true positives. Thus, the chance a positive result is indicating prostate cancer is 11/16 or 68%.